The heart has four chambers. The upper two are the right and left atria. The lower two are the right and left ventricles. Blood is pumped through the chambers, aided by four heart valves. The valves open and close to let the blood flow in only one direction.

What are the four heart valves?

• The tricuspid valve is between the right atrium and right ventricle.
• The pulmonary or pulmonic valve is between the right ventricle and the pulmonary artery.
• The mitral valve is between the left atrium and left ventricle.
• The aortic valve is between the left ventricle and the aorta.

Each valve has a set of flaps (also called leaflets or cusps). When working properly, the heart valves open and close fully.

Heart valves don't always work as they should. A person can be born with an abnormal heart valve, a type of congenital heart defect. Also, a valve can become damaged by

• infections (e.g. infective endocarditis)
• changes in valve structure in the elderly
• rheumatic fever

A mechanical (or artificial) heart valve is a man-made device that is used to replace one of a patient’s own damaged or diseased heart valve that cannot be repaired. A biological valve, from either an animal (xenograft) or a deceased human donor (allograft) may also be used to replace the patient’s original valve.
In most cases, the use of a mechanical heart valve can lengthen or even save a patient’s life. The valves are durable and can last 30 years or longer. However, there is a risk of complications, and most patients will need to take anticoagulants for the rest of their lives to reduce the risk of blood clot formation.
Here we perform a numerical study of a valve construction based on a curved central guide strut and a flat disc. This has two advantages: (i) It allows assembly of the valve and disc without imparting stress on the valve housing and (ii) it allows the disc to move out of the annular plane (which is the tightest constricture of the resultant outflow tract). This type of valve is produced by Medtronic.

Turbulence in the cardiovascular system leads to higher flow resistance, resulting in increased pressure gradients. Furthermore, elevated levels of turbulent shear stresses may create hemolysis or platelet activation [1], [5]. This may in turn lead to thrombosis [8] and embolism. It was also shown that turbulent shear stresses can be associated with the development of aortic aneurysms [3], [6].
The damage done to red blood cells can be described as a function of spatial distribution, exposure time and magnitude of turbulent shear stresses. In particular, in [7] and [9] the critical parameters of turbulent shear stress were identified, which lead to lethal or sublethal damage of blood cells.
The goal of an optimal heart valve is to retain a near physiologic turbulence profile. The benefits are minimal pressure gradients and very low levels of thrombosis and thromboembolism. This was experimentally prooved for the Medtronic Hall valve in [2].
To achieve this design goal, computational fluid dynamics simulations are of great advantage. The valve and the adjacent arteries were modeled using NURBS to facilitate (i) adaptive mesh refinement and (ii) a parametric geometrical model which is suitable for design optimization. The fluid zone was meshed automatically by a hexahedral mesh using approx. 400.000 elements. The fluid problem was modeled using a non-Newtonian model which was solved using a modified SIMPLE algorithm. The moving bodies were considered using fluid structure interaction considered. Thereby, the arterial wall is simulated as a hyperelastic medium and the valve disc as a rigid, but movable body. The results of the simulation are shown in Fig. 1 and 2.

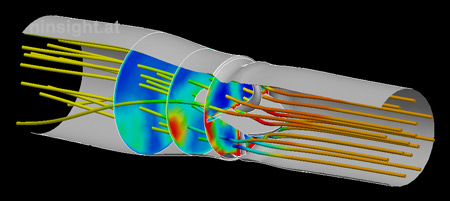Fig. 1: CFD simulation of the mechanical heart valve. The image depicts the flow situation at the systolic phase (the valve is fully opened under 75°). Streamlines are colored by the local pressure. Section planes show the turbulent dissipation.

Despite a common belief in a symmetrical (i.e., bullet-shaped) flow pattern in the ascending aorta, research has confirmed that natural aortic flows are eccentric—with the region of highest velocity occurring in the non-coronary sinus [Paulsen et al.].

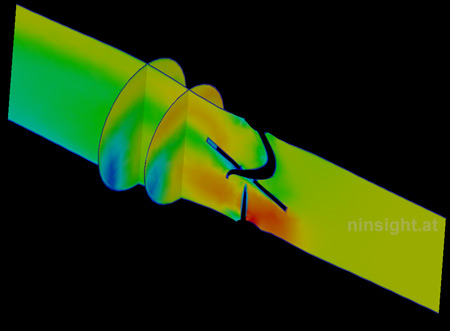Fig. 2: Velocity magnitude at fully opened valve (three representative sections).

1. Kroll MH, Hellums JD, McIntyre LV, et al. Platelets and Shear Stress. Blood 1996;88:1525-41.
2. Kleine P, Perthal M, Nygaard H, et al. Medtronic Hall versus St. Jude Mechanical Aortic Valve: Downstream Turbulences with Respect to Rotation in Pigs. J Heart Valve Dis 1998;7:548-55.
3. Nichols WW, O'Rourke MF. McDonald's Blood Flow in Arteries. Theoretic, Experimental, and Clinical Properties. 3rd ed. Philadelphia: Lea & Febiger, 1990;54-71.
4. Paulsen PK, Nygaard H, Hasenkam JM, et al. Analysis of Velocity in the Ascending Aorta in Humans. A Comparative Study Among Normal Aortic Valves, St. Jude Medical and Starr-Edwards Silastic Ball Valves Int. J Artif Org 1988; 11:293-302.
5. Ruggeri ZM. Mechanisms of Shear-Induced Platelet Adhesion and Aggregation. Thromb Haemost 1993:70:119-123.
6. Stein PD, Sabbah HN. Hemorheology of Turbulence. Bioheol 1980;17:301-19.
7. Tillmann W, Reul H, Herold M, et al. In Vitro Wall Shear Measurements in Aortic Valve Prostheses. J Biomech 1984;17:263-79.
8. Yoganathan AP, Wick TM, Reul H., The Influence of Flow Characteristics of Prosthetic Heart Valves on Thrombus Formation. I: Butchart EG, Bodner E (eds.) Current Issues in Heart Valve Disease: Thrombosis, Embolism and Bleeding. London: ICR, 1992;123-48.
9. Yoganathan AP, Woo Y-R, Sung H-W. Turbulent Shear Stress Measurements in Aortic Valve Prostheses. J Biomech 1986;19:433-42.